Technical Note No. 2000/1 - Conversion of Hydrostatic-Depth Spectrum to Sea-Surface-Elevation Spectrum
Since the release of version 19.5, PEDP reports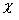 
beside each spectral estimate Sh(f) when processing Task 4/1 spectra.
is defined as:
where z* is the depth of DOBIE below mean water level, 
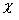is the mean water depth and k is the wavenumber corresponding to f, the frequency of the spectral estimate. k is computed using linear wave theory.) Note that is the reciprocal of the "penetration" that has been defined in previous Technical Notes, and is therefore always greater than or equal to 1.
Sh(f) is the spectrum of the "hydrostatic depth", h(t), as explained in Technical Note 98/6, which is simply pressure converted to a depth using the hydrostatic equation. This is an essentially meaningless quantity on its own(!), since it is not pressure anymore, it is not really depth and, even when you subtract mean depth from it, it is not sea-surface elevation around mean water level, either. The reason is, as explained in Technical Note 98/1, that pressure under waves is not exactly hydrostatic and therefore using the hydrostatic equation to convert pressure to depth is not exactly correct.
Calculating Sh(f) is, however, the first step towards estimating Sh(f), which is the sea-surface-elevation spectrum, because:
corrects each frequency component of the wavetrain for the decrease in amplitude of pressure fluctuations below mean water level. The attenuation varies with frequency (it is greater for the higher frequency components), which is why 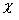must be calculated for each component of the spectrum. Having obtained Sh(f), it may be inverse-transformed to obtain 
, the time series of sea-surface elevation. then may be subjected to wave-by-analysis or bulk statistical analyses in the usual ways.
There always seems to be a flaw somewhere, and this is it for this procedure: 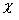quickly gets very big as f increases and, at the same time, the signal-to-noise ratio in Sh(f) very quickly decreases. The result is that noise in the high-frequency tail of the hydrostatic-depth spectrum gets amplified by the ever-increasing values of 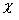and so the high-frequency components of Sh(f) get ridiculously overestimated. The trick is knowing when to stop multiplying by 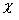. There are theoretical and empirical methods available for predicting the cutoff, but for now, PEDP leaves that to you, the user.
MOG May, 2000